|
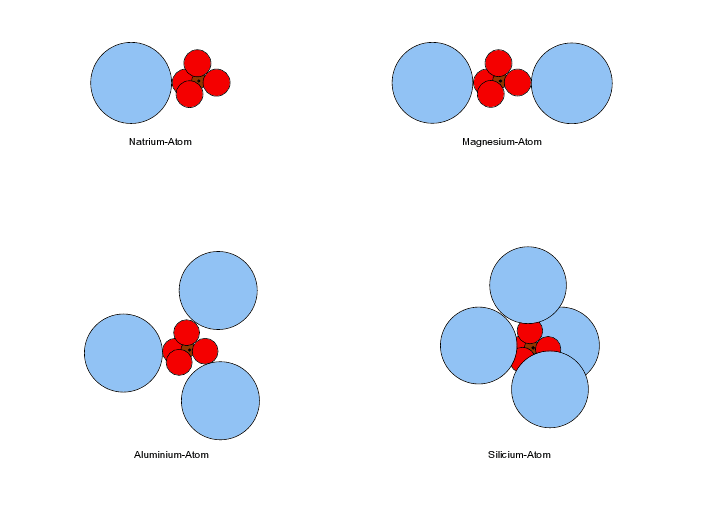
Na | single cloud in face of [Ne] tetrahedron |
|
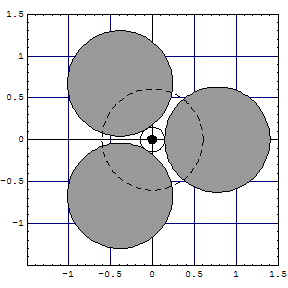
Mg | two single clouds at 180° touch [Ne] tetrahedron along C2 axis (figure) |
|
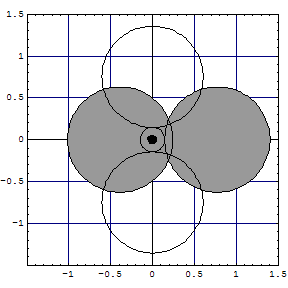
Al | three clouds in D3h touch tbp deformed [Ne] tetrahedron (figure) |
|
Si | Td |
|
P | Td single, double occupation same radius, should be -> C3v |
|
S | Td single, double occupation same radius, should be -> C2v |
|
Cl | Td single, double occupation same radius, should be -> C3v |
|
Ar | Td |
|
K | single cloud in face of [Ar] tetrahedron (figure)
|
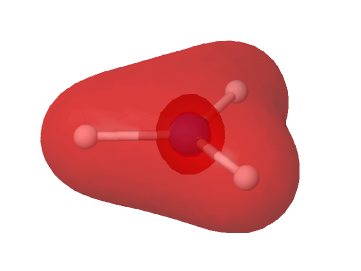
These computations make it clear, e.g. with Mg, Al, and K, that the "construction" of an atom with more than two shells of nonoverlapping spheres needs symmetry adjustments. These are far from artificial, since e.g. the Al atom really forms a D
3h AlH
3 and AlF
3 molecule, which have a trigonally deformed Ne core. The plot for K marks the end of a painstaking stacking of tetrahedra ("qualitative" Kimball fans have claimed at several places that Kimballs model cannot be used beyond K (or even Ar) for this reason). A continuation by tetrahedra brings us at variance with the Periodic Table, since we have to accommodate the 10 3d electrons before we can complete the 4s
24p
6 shell at Kr. That can be done by abandoning tetrahedral for a trigonal-bipyramidal arrangement up to Ni or Zn. We will do so in the
spherical atoms but first fuse the inner tetrahedra to a common, energy equivalent outer sphere, each, centered at the nucleus.
Except for
Al all the above atoms have a tetrahedral [Ne] core.
Al needs a trigonal deformation for the D
3h arrangement of three half filled valence clouds. We have used a deformed Ne core with trigonal bipyramidal form. Let's compare the two Ne cores to give us an idea of how much we may manipulate the 2s
2p
6 density packed into Kimball spheres:
| Ne core | Etot | R1 |
R2 | R3 axial | Vne | Vee | Virial |
|
Td | -128.908 | 0.145796 |
0.624762 | | -309.588 | 51.7725 | 2.00000 |
tbp | -128.775 | 0.145963 |
0.629791 | 0.604871 | -309.512 | 51.9613 | 2.00000 |